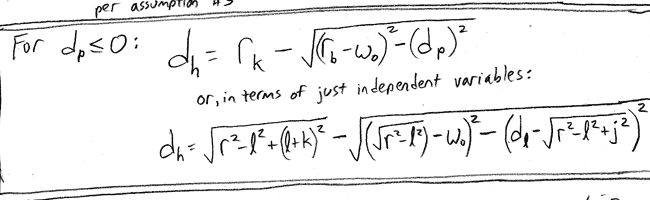Perpendicular Parking Mathematics (Nov 20, 2010; updated Dec 12, 2010)
Back to mathematics of parking page
Inspired by the news story of late last year, British Mathematician Perfects Parallel Parking (Dec 15, 2009), I decided to tackle the mathematics of parking perpendicular to the path of travel. Like my explorations last year, I searched for an analytic solution in the form of algebraic formulas and also created interactive animations with the free Geogebra mathematics software. Unlike last year's explorations, however, I concede that my models may not represent the ideal/perfect parking geometry. I'd claim that they at least come close to determining the smallest required lateral space for perpendicular parking (forward motion only) while keeping the geometry relatively easy to analyze (line segments and arcs). The British mathematician, Professor Blackburn, claims in his original report that the optimal geometry would involve the much more complicated "tractrix" curve. I initially was inclined to agree. The algebra/calculus involved in analyzing a tractrix model looked too ambitious for me to tackle at this time, but I set out to see if I could make a Geogebra model based on this curve in a reasonable amount of time. I pretty quickly concluded that following such a path would require an unrealistically tight turning radius at the start of the turn, and therefore the tractrix alone doesn’t represent a reasonable real-world solution.
So what is the optimal solution? Perhaps a combination of arcs, line segments, a tractrix, and/or some other curve I haven’t considered? I don’t pretend to know. Just looking at the Geogebra models below though (and keeping in mind the setting for minimum turn radius), I believe that the optimal solution could only buy a few more inches/centimeters at best.
In the Geogebra/Java files embeded below, drag the sliders to vary each of the following parameters. All distances are represented in feet for the US customary version, or meters for the metric version. Press the pause button in the lower left corner to stop the animation. Hold the shift key and then drag to pan left/right/up/down.
- j: distance from rear axle to rear bumper
- k: distance from front axle to front bumper
- l: wheel base (distance between front and rear axles)
- r: curb-to-curb turning radius
- w_0: width of car being parked
- d_l: lateral distance available, perpendicular to the path of travel
- d_h: minimal distance between two cars parked perpendicular to the path of travel (dependent variable to be solved for)
As the values of the parameters are modified, note that the cars parked on each side adjust automatically as necessary to avoid contact during the course of the turn. This hints at the "piecewise" nature of the algebraic solution.
It may take a moment for the Java applet to load below. Go ahead and "accept" if prompted. The alert sounds threatening, but it's safe. Dude, trust me.
Geogebra model in US Customary Units
Geogebra model in Metric Units
Algebraic Solution
Depending on the location of the center of rotation relative to the parked cars, the "piecewise" formula takes one of three forms. Like Professor Blackburn's formula, the heavy dependence on repeated usage of the Pythagorean Theorem is quite evident.
Click on image below to see the formulas and their entire derivation.
Jerome A White, November 2010, Created with GeoGebra